What so far?
Just a quick review of what we discussed earlier, number of protons in the nucleus determines the identity (element) of the atom, and number of neutrons determines the isotopes and physcial properties of the atoms.
In this post, we'll focus on the most important physical property, mass, of the atom.
Abundance
Since both proton and neutron have a mass of 1 amu (as discussed in this post), mass of an atom would be the same of its mass number with the unit amu because mass number is defined as the total number of protons and neutrons, still remember?
For example, if there is an atom of isotope chlorine-37, (symbol: \( \ce{^37Cl} \)), it would have a mass of 37 amu, whereby \( 1 \mathrm{amu} = \pu{1.6726219E-27} \mathrm{kg} \). For the other common isotope of chlorine, chlorine-35 (\( \ce{^35Cl} \)), it has a mass of 35 amu.
| Isotope | \( \ce{^35Cl} \) | \( \ce{^37Cl} \) |
|---|---|---|
| Mass | 35 amu | 37 amu |
| Abundance | 75% | 25% |
Common isotopes of chlorine
If we grep a handful of chlorine atoms and count the number of \( \ce{^35Cl} \) and \( \ce{^37Cl} \) atoms we get, we'll find that 75% of the total chlorine atoms would be \( \ce{^35Cl} \), while the rest are \( \ce{^37Cl} \) (25%). This is called abundance.
Do take note that the abundance does not change with the source where we get the atoms from. You may find chlorine atoms in swimming pools, as people use chlorine for disinfection there; you can also find chlorine atoms in table salt which is actually the source of chlorine in industry. But it doesn't really matter whether you get chlorine atoms from swimming pool or table salt, you'll always find 75% out of the total chlorine atoms being \( \ce{^35Cl} \) and the rest being \( \ce{^37Cl} \).
Because of that, we may simply calculate an average mass of chlorine atoms to represent all isotopes rather than mentioning the mass of a specific isotope each time. That is the atomic mass.
Atomic mass
Atomic mass is the weighted average of masses of all naturally occuring isotopes of an element based on their abundance. Let's do a simple math here.
Again, let's use the chlorine atoms as the example. Image there are 100 chlorine atoms, no matter where we get them, 75 atoms will be \( \ce{^35Cl} \), and 25 atoms will be \( \ce{^37Cl} \).
Since \( \ce{^35Cl} \) has a mass of 35 amu, 75 \( \ce{^35Cl} \) atoms have mass of
\[ 75 \times 35 \mathrm{amu} = 2625 \mathrm{amu} \]
We can do the same to those \( \ce{^37Cl} \) atoms. 25 \( \ce{^37Cl} \) atoms will be
\[ 25 \times 37 \mathrm{amu} = 925 \mathrm{amu} \]
Therefore, the 100 chlorine atoms will have a total mass of
\[ 2625 + 925 = 3550 \mathrm{amu} \]
So in average, each chlorine atom has a mass of
\[ \frac{3550}{100} = 35.50 \mathrm{amu} \]
We can now say that we have 100 chlorine atoms and all of which have a mass of 35.50 amu. See? We no long worry about whether we have \( \ce{^35Cl} \) or \( \ce{^37Cl} \). We can consider all chlorine atoms are identical and they all have an atomic mass of 35.50 amu.
In short, we could calculate atomic mass of an element by this formula
\[ atomic\ mass = \sum (abundance \times mass\ number) \]
So for chlorine, we can simply do
\[ atomic\ mass = 75\% \times 35 + 25\% \times 37 = 35.50 \mathrm{amu} \]
Try yourself
What is the atomic mass of boron (B), given that it has two isotopes \( \ce{^10B} \) and \( \ce{^11B} \), which has an abundance of 80% and 20% respectively?
Relative atomic mass
We have shown how to calculate atomic mass of an element if we know its isotopes and their abundance. Atomic mass will have a unit of amu, which is equal to the mass of a single proton or neutron.
Since all atoms are measured against the same standard (1 amu), sometimes we would care more about the values of their atomic masses, rather than the unit.
Therefore, we would more commonly refer to another concept relative atomic mass, which is defined as the ratio between atomic mass and \( \frac{1}{12} \) of mass of a single \( \ce{^12C} \) atom.
So let's do a simple math here. Just now we have already calculated the atomic mass of chlorine is 35.50amu. Now we shall see what is happening if we divide that by \( \frac{1}{12} \) of mass of a single \( \ce{^12C} \) atom.
As we have studied from the previeous posts, \( \ce{^12C} \) has a mass number 12, which indicates a mass of 12 amu. \( \frac{1}{12} \) of 12 amu would simply be \( \frac{1}{12} \times 12 \mathrm{amu} = 1\mathrm{amu} \).
Therefore, \( \frac{35.50\mathrm{amu}}{1\mathrm{amu}} = 35.50 \) without any unit.
Relative atomic mass has the same value as atomic mass, but without a unit.
We use relative atomic mass more often because we do not really care about the mass of a single atom. Anyway, even with atomic mass, we are still unable to tell the actual mass of an atom directly because the unit is not in grams. What matters for us as chemists is the difference between the masses of different atoms. Therefore, we would simply use relative atomic mass to tell us that difference and we would not bother its unit.
For example, the relative atomic mass of \( \ce{C} \) is 12.01 while that of \( \ce{O} \) is 16.00. This tells us that a single carbon atom has an average mass that is 12.01 times as \( \frac{1}{12} \) of mass of a single \( \ce{^12C} \) atom; and a single oxygen atom has an average mass 16.00 times as \( \frac{1}{12} \) of mass of a single \( \ce{^12C} \) atom. So oxygen is having a mass \( \frac{16.00}{12.01} \) times as that of carbon.
In one word, we use relative atomic mass to represent the average atomic mass of an element. It takes into consideration the abundances of different isotopes of that element. It is a unitless number and allows us to compare the mass difference between elements; but does not directly tell us the mass of a single atom (which we do not really want to know).
Periodic table again
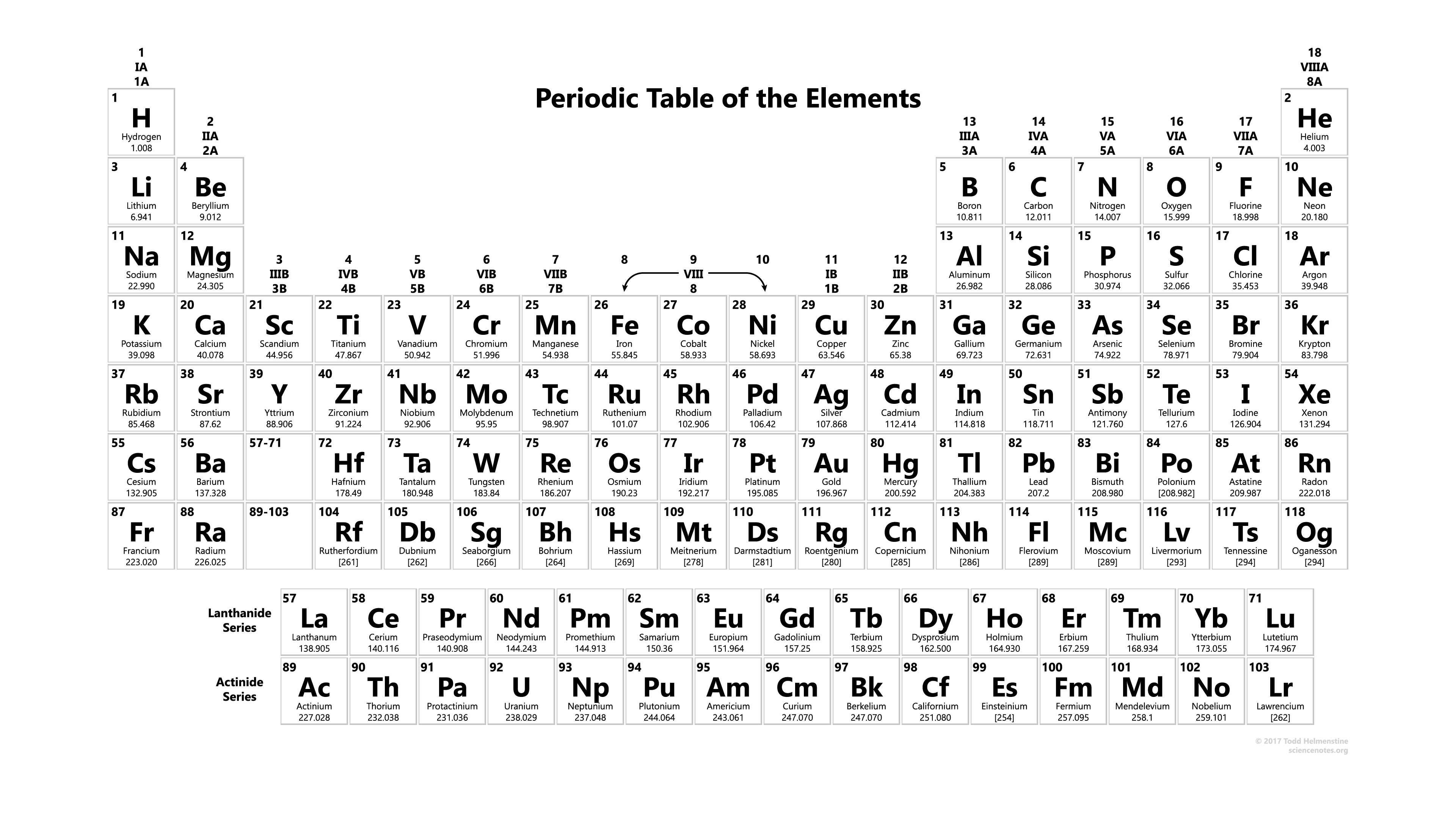
Again, scientists have already done all the experiments for us, and they have included relative atomic mass of all the elements in the periodic table.
As you may see from the periodic table above, the number at the bottom of each box shows the relative atomic mass of that element. So next time if someone asks you what is the relative atomic mass of aluminum (\( \ce{Al} \)), you would not say "Wait, let me do some experiments to figure that out". But rather, you can take out your periodic table, and tell him "It's 26.982"!
Periodic table is one of the most important tool for chemists. So be familiar with it, and more importantly, make good use of it.